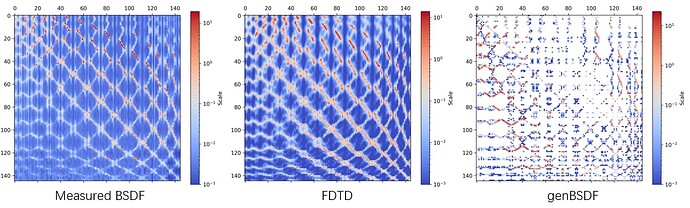
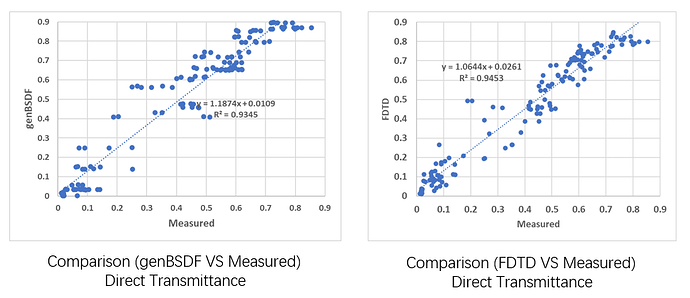
I use a computational electromagnetic method (FDTD) to generate the BSDF for a prismatic structure with a 50 µm periodic length and compare it with those calculated using genBSDF and measured BSDF. The BSDF generated with FDTD shows better consistency with the measured one. However, because I only have one measured BSDF for the prismatic structure, I cannot compare more structures to verify the accuracy level of the proposed method. So I want to ask if anyone would like to share your measured bsdf data for a micro-nano materials, so we can work together to do the research. If anyone have interest, please contact me (My email: [email protected]).Note: Finite-difference time-domain (FDTD) is a method for solving Maxwell’s Equations, which describe classical Electrodynamics.
Very interesting work!
It looks like both methods have outliers, likely due to differences between the idealized model and the actual material, which has no doubt some deviations due to manufacturing. In particular, corners are unlikely to be perfect, and this leads to significant light leakage.
In general, I would expect the FDTD method to perform much better when important geometric details are in the < 5 micron range, since ray optics do not perform well for visible light with details approaching the considered wavelength. I know you know this.
Finally, are you modeling dispersion – how the substrate’s index of refraction varies with wavelength? I would think this important for a general study of prismatic systems. Radiance does not model this property very well, so you would need to make multiple runs with different refractive indexes to apply genBSDF with dispersion.
Cheers,
-Greg
Hi Greg,
Thank you for providing me with such important information.
I obtained the complex refractive index from this site: Refractive index of (C10H8O4)n (Polyethylene terephthalate, PET) - Zhang. It contains the complex refractive indices for various wavelengths. However, so far, I have only modeled the BSDF for a specific frequency point and haven’t accounted for dispersion. This is because the size of the modeled prism (50 microns) is quite large, resulting in very long computation times. In practice, ray tracing might be sufficient for such a large structure since diffraction is not as significant, which diminishes the advantages of FDTD. However, I believe the method is not only suitable for the prism but also for other micro- and nano-structures. The issue is that I don’t have measured BSDFs for smaller structures, so I wanted to ask if anyone could share their data.
Regarding broadband FDTD simulations, the Broadband Fixed Angle Source Technique (BFAST) can be used. However, the simulation time increases with the incident angle. When the incident angle reaches 80°, the simulation time increases by 65.8 times. Therefore, at grazing angles, multiple runs with bloch boundaries might be faster.